To learn Bode diagram today, for many closed-loop systems, we need to draw its Bode diagram to analyze its stability, and the quality of a system's dynamic and static performance can be intuitively reflected from the Bode diagram. Before explaining the Bode plot, we first review the complex knowledge. The following figure shows the complex plane, that is, the coordinate system with the real part as the x-axis and the imaginary part as the y-axis. Given a complex number, we can find its modulus and amplitude using the following two formulas, and the definition of the decibel concept is the logarithm to the base 10 of the 20-fold gain. The reason why the logarithm is selected here is that it can include a larger frequency range with limited coordinates.

Next, look at the Bode plot of the first-order system. First, give the transfer function of the first-order system, and take its modulus. Here, the modulus of the fraction is equal to the modulus and the denominator of the numerator, and then divided and then the logarithm is obtained. In the decibel form of the gain, where the DC gain is 0 dB, the angle can also be obtained. The bottom right picture is the Bode diagram of the second-order system, but we can only look at the low-frequency part (<100 kHz) when the frequency is very low (< 1Hz), the system gain is a DC gain, and for an RC first-order filter system, the DC gain is 0dB, and when the frequency is greater than the first pole, the gain is decreased by -20dB per decade and the angle is -45 degrees. , will be reduced to -90 degrees afterwards.

Next, we will explain the Bode diagram of the second-order system. Similarly, let's review the knowledge of mathematics first. Using Euler's formula can simplify the multiplication and division of complex numbers. Observing the following formula, we can see that the complex multiplication, the result of the module As a product of the modulo of two multipliers, the resulting argument is the sum of the two multiplier arguments. In the same way, the division of plural numbers is divided by their moduli and their amplitudes are subtracted.

In this way, we will be more convenient in calculating the second-order system's mode and amplitude. We first factorize the numerator denominator of the transfer function to get the following equation and then take the modulus. Here, we can use the division relationship first. The quotient is then the multiplication relationship, so that the mode is the mode of each factor, and the angle is the same, and the sum of the argument angles of each factor is subtracted from the argument of each factor of the denominator. In this way, you can draw the Bode plot and look at the Bode plot on the right. After the first pole, the gain is decreased by -20 dB per decade (in the figure, -6 dB is decreased by each multiplier, but the rate of decline is the same ), the phase angle drops to -45 degrees and then drops to -90 degrees at infinity. After the second pole, the gain decreases by -40 dB per decade, the phase angle drops to -135 degrees, and then infinity The distance drops to -180 degrees. To sum up, the effect of the pole is to change the slope after the pole frequency above the gain. After each pole, the rate of gain reduction increases by -20 dB per decade, and in the phase angle, the change in the phase angle is gradually generated. The specific embodiment is that after each pass of a pole, the angle gradually decreases by 45 degrees from the previous angle, and then gradually decreases by 45 degrees. The zero point is similar. Its effect is that, after the gain, the slope after the zero frequency is changed, and the rate of increase of the gain is increased by 20 dB per decade after every zero point. In the phase angle, the change of the phase angle is gradually generated. This is reflected in that after each pole is passed, the angle is increased by 45 degrees from the previous angle, and then gradually increased by 45 degrees. It can be seen that the pole-zero effect is reversed, so the zero or pole is added to offset the pole or zero to correct the system.

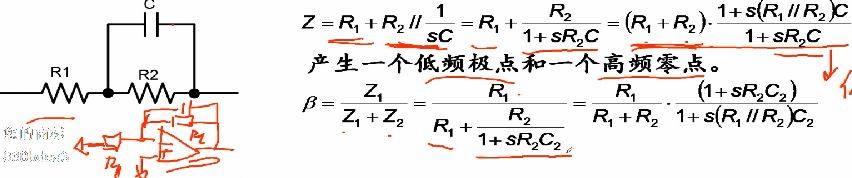
A pole is created when the resistor and capacitor are connected in parallel.

When the resistors and capacitors are connected in series, they produce a zero and zero pole.

The following analysis of the Bode diagram of the circuit below is also a commonly used form of the op amp circuit. The first analysis is from the perspective of impedance, and a low frequency pole and a high frequency zero point will be generated. The definition of zeros and poles here is to make the solution of each factor zero S, and then analyze it from the perspective of feedback, resulting in a high frequency pole and low frequency zero point.
DADNCELL 1.5V AAA alkaline batteries are more suitable for long-lasting continuous discharge and high-performance power consumption equipment because of their unique internal component structure, coupled with better battery materials, improved power capacity and electrical performance, and the same model of DADNCELL 1.5V AAA alkaline cell capacity and discharge time, Alkaline battery capacity and discharge time are 4-7 times that of ordinary batteries, and the gap in power discharge performance at high and low temperature is even wider.
At present, all types of cells developed by DADNCELL Labs do not involve any heavy metals in production, are green and can be disposed of with domestic waste.
DADNCELL 1.5V AAA battery advocates a more comfortable and smooth power supply experience for household appliances. Alkaline zinc-manganese dry battery series is suitable for various common household electronic equipment instruments such as smart door locks, infrared thermometers, cameras, flash lights, razors, electric toys, instruments, high-power remote controls, Bluetooth wireless mouse keyboards, etc.
1.5V Aa Alkaline Batteries,1.5V Aa Alkaline Batteries Suitable For Infrared Temperature Gun,1.5 Aa For Camera Instruments,1.5V Lr6 Alkaline Batteries Suitable For Intelligent Door Locks
Shandong Huachuang Times Optoelectronics Technology Co., Ltd. , https://www.dadncell.com